Generating random permutations

Generating permutations given a list of items is a simple problem which can be solved with a few lines algorithm. Anyway it is also one of the problems that people often get wrong.

Given a set of items

generating a random permutation of the elements mean giving each possible permutation (i.e. each one of the \( n! = 3! = 6 \) possible permutations) the same probability of being generated.

The simplest algorithm that might come to mind is the following

for(int i=0; i<N; ++i) {
  perm[i] = arr[i];
}

for(int i=0; i<N; ++i) {
  int rand = random(0, N); // Random number in [0;N[
  swap(arr[i], arr[rand]);
}

The algorithm above is incorrect and fails to deliver a uniform distribution for all the permutations of a given set. The reason why this algorithm is incorrect lies in the way permutations are generated.

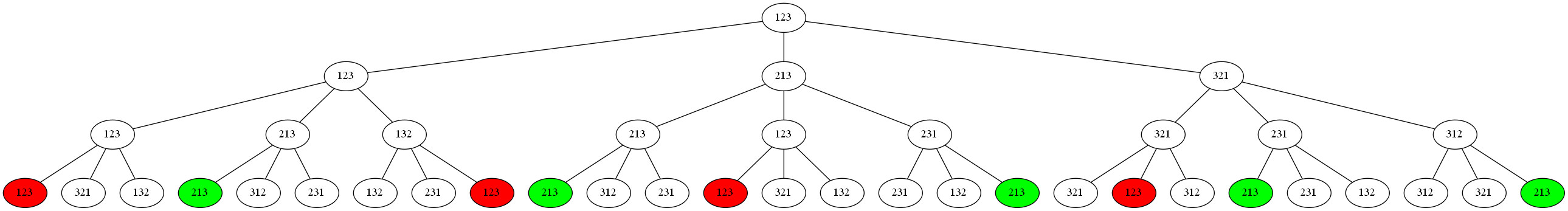
Let’s take, for instance, the complete tree for the previous given set of elements (click the image to enlarge it)

The final nodes which yield the \( 1,2,3 \) permutation have been colored in red, while the nodes for \( 2,1,3 \) have been colored in green.

Every edge has a probability of \( \frac{1}{3} \) and thus probabilities are

Therefore this is not a uniform distribution of probabilities for random permutations.

A better algorithm is

for(int i=0; i<N; ++i) {
  perm[i] = arr[i];
}

for(int i=0; i<N; ++i) {
  int rand = random(i, N); // Random number in [i;N[
  swap(arr[i], arr[rand]);
}

Let’s calculate the probability of generating the permutation \( {1,2,3} \): the probability for the first element is \( \frac{1}{3} \), while the probability for the second number is \( \frac{2}{3} \cdot \frac{1}{2} = \frac{1}{3}\) i.e. the probability of not being extracted as first number and the probability of being extracted in the second position. The third element’s probability is, similarly, \( \frac{2}{3} \cdot \frac{1}{2} = \frac{1}{3}\). Every element has probability \( \frac{1}{3} \) and thus this is a uniform distribution.